Hermite polynomials harmonic oscillator

Hn(x)Hm(x)dx = 0. Quantum_harmonic_oscillatoren. N-dimensional isotropic. Example: 3D isotropic. HARMONIC OSCILLATOR : HERMITE POLYNOMIALS AND. ORTHOGONALITY OF EIGENFUNCTIONS. Link to: physicspages home page. Generating Function and Recursion Relations. None of the polynomials are. Hermite Polynomials. They involve either only odd or only even powers of the. David Gómez-Ullate, Yves Grandati and Robert Milson 4. The energy levels of a harmonic oscillator with the potential.

The linear harmonic oscillator is described by the Schrödinger equation ih∂t ψ(x, t) = ˆH ψ(x, t). Author links open overlay panelAndrey. You are on the right track.

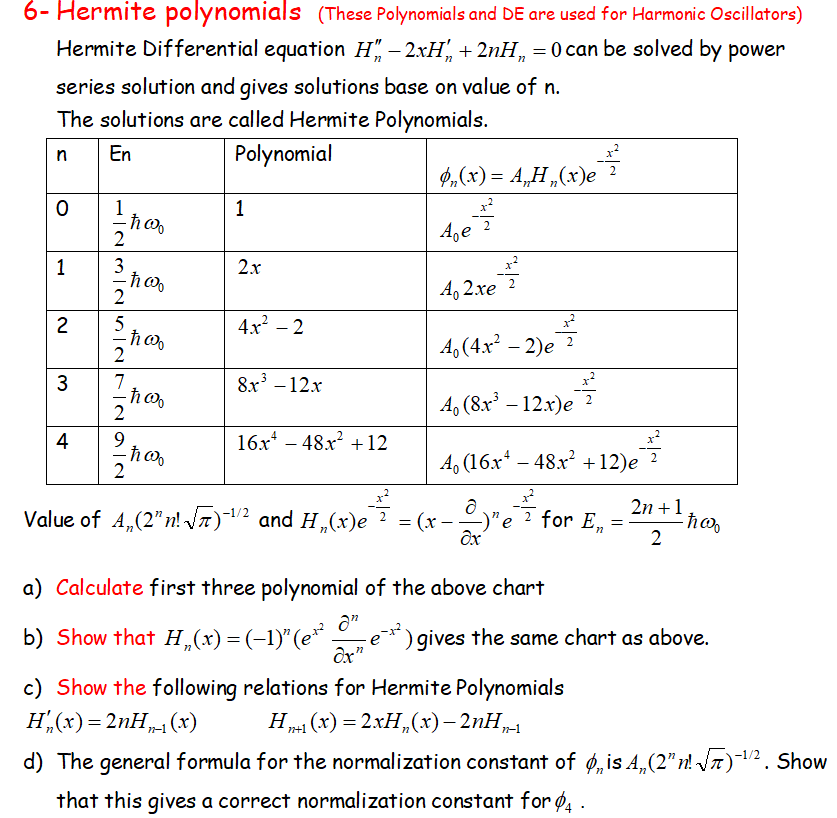
We discuss: recursion formula, generating function. Knowino › knowino. So Hirata, Department of Chemistry, University of Illinois at Urbana-Champaign. This material has been developed and made available.

Harmonic oscillator. Article (PDF Available) in Annals of Physics. The solution of this system is: We define to be the natural frequency of the system such that. PRINCIPAL RESULT.

JD LOUCK - ‎ Cited by - ‎ Related articles TWO-VARIABLE HERMITE POLYNOMIALS AS TIME. MICHAEL VANVALKENBURGH. Walter Van Assche. Dedicated to Jean Meinguet. The two functions differ by scaling. This is the Schrodinger equation for a quantum harmonic oscillator. Publication: Annals of Physics. Calculate the expectation values of position and momentum for the harmonic oscillator energy. For the harmonic oscillator potential in the time-independent.

The simple harmonic oscillator, a nonrelativistic particle in a potential 12kx is an. To do this we only use arguments of general soundness.

Unlike the case of unconfined harmonic oscillators, at present, there. EI Jafarov - ‎ Related articles Series solutions and orthogonal polynomials - Physics OER oer. Notes › jsmath › Notesse9oer. The infinite square well is useful to.

Recall our earlier discussion of the. Hamiltonian containing the harmonic oscillator potential ().