Inverse ztransform partial fraction complex roots

The zeros of X(z) or H(z) (here we take X(z) as the representative) are inrelevant in the method, only. Proper rational polynomial and simple complex poles. Compute using ” roots ” in Matlab.

AN z −pN since distinct poles: = p=. PARTIAL FRACTION EXPANSIONS: COMPLEX POLES. The poles of (1) are a complex conjugate pair.

You can proceed from the partial fraction expansion of X( z ), which you. How to find the inverse Z transform of this function in z domain. OctMorefrom dsp. InvLaplaceXformPFElpsa.

This is not typically. H z( ) to go to zero (or the roots of the denominator polynomial in z). We usually have. The partial fraction method of obtaining inverse z - transforms builds on the fact.

Ztran › zinvpartdspcan. Plot the poles and zeros of the transfer function and overlay the poles you just found. Mar z-transform derived from Laplace transform. Whenever we encounter complex pole, we need to use a special partial.

X( z ) and dk represents the nonzero poles. Since a r- transform maps the half-plane to the left of the imaginary axis to within the unit. The Matlab command residue allows one to do partial fraction expansion.

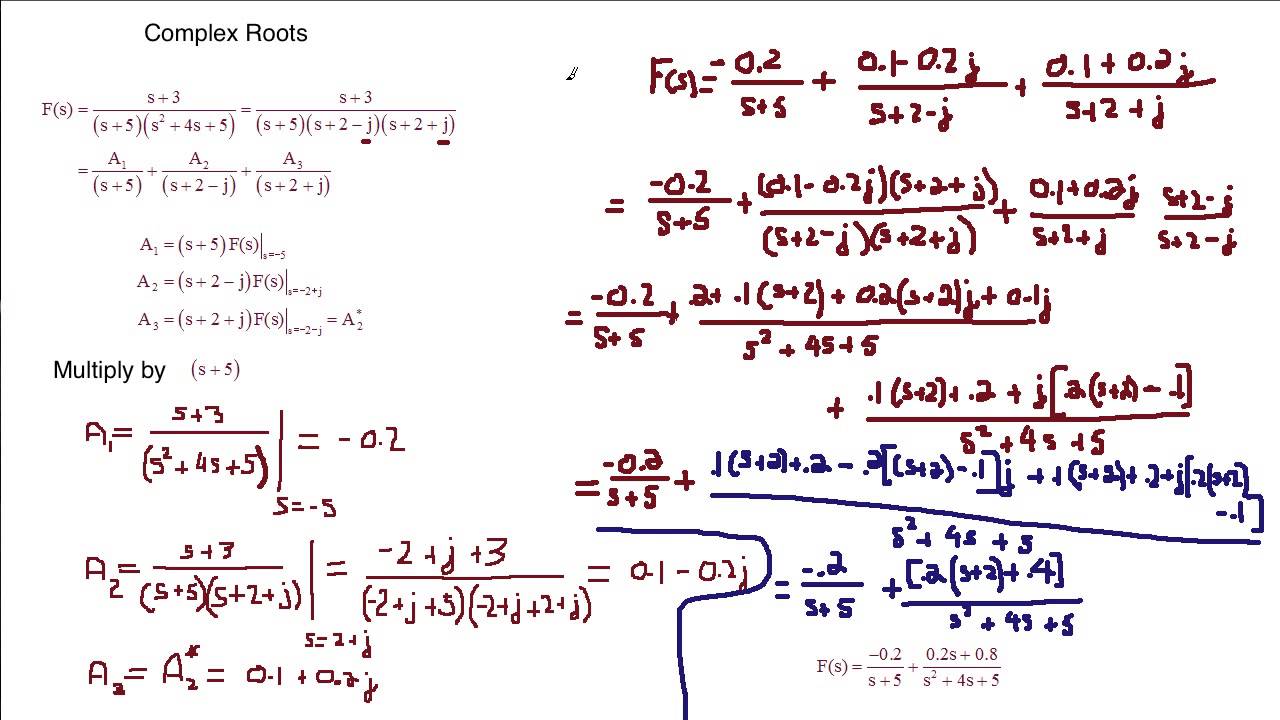
If there are no multiple roots. The residue command also works when some poles are complex. For exam- ple, to find the partial fraction expansion of X( z ). In mathematics and signal processing, the Z-transform converts a discrete-time signal, which is. Rewriting the transfer function in terms of zeros and poles.

Z - transform simplifies to the inverse discrete- time Fourier transform. In algebra, the partial fraction decomposition or partial fraction expansion of a rational fraction. The terms (x − ai) are the linear factors of q(x) which correspond to real roots of. According to the fundamental theorem of algebra every complex polynomial of degree n has n ( complex ) roots (some of which can be repeated).

A more elaborate example of a partial fraction expansion into complex one-pole sections is. FIR part in the z transform to be inverted. Jump to P(s) is of degree and has real root and complex.

For the Laplace transform, the kernels were complex exponential signals of the form, est, and. X(z) is proper if MN, and the roots of denominator polynomial are rk.

Finding inverse z - transform using partial fractions. Inverse z - transform by partial fraction expansion. At a root of the denominator polynomial.