Quantum harmonic oscillator

Because an arbitrary smooth potential can usually be approximated as a harmonic potential at the vicinity of a stable equilibrium point, it is one of the most important model systems in quantum mechanics. N-dimensional isotropic. Example: 3D isotropic. A diatomic molecule vibrates somewhat like two masses on a spring with a potential energy that depends upon the square of the.

It can be applied rather directly to the explanation of the vibration. Professor Allan Adams. Massachusetts Institute of Technology. Assigned Reading.

A simple harmonic oscillator is a particle or system that undergoes harmonic motion about an equilibrium. Quantum harmonic oscillator (QHO) involves square law potential (x2) in the Schrodinger equation and is a fundamental problem in quantum.

This is the first non-constant potential for which we will solve the Schrödinger Equation. A harmonic oscillator ( quantum or classical) is a particle in a potential energy well given by V(x)=½kx².

At sufficiently small energies, the harmonic oscillator as governed by the laws of quantum mechanics, known simply as the quantum harmonic oscillator, differs. In passing from a classical treatment to a quantum mechanical one, the dynamical variables are replaced by operators x $ Χ. A study of the simple harmonic oscillator is important in classical mechanics and in quantum mechanics.

Comparison of the classical and quantum harmonic oscillator. Consider a quantum particle of mass m confined to a one-dimensional region of potential energy. Zero Normalize Coherent(α) α = 1. We investigate the dynamics of the spin-less relativistic particle subject to an external field of a harmonic oscillator potential.

In figurethe dark solid curve shows the average energy of a harmonic oscillator in thermal. This is a standard piece of theory in quantum mechanics, and it is crucial for.

Abstract: A correspondence is established between measure-preserving, ergodic dynamics of a classical harmonic oscillator and a quantum. It comprises one of the most important examples of elementary Quantum Mechanics.

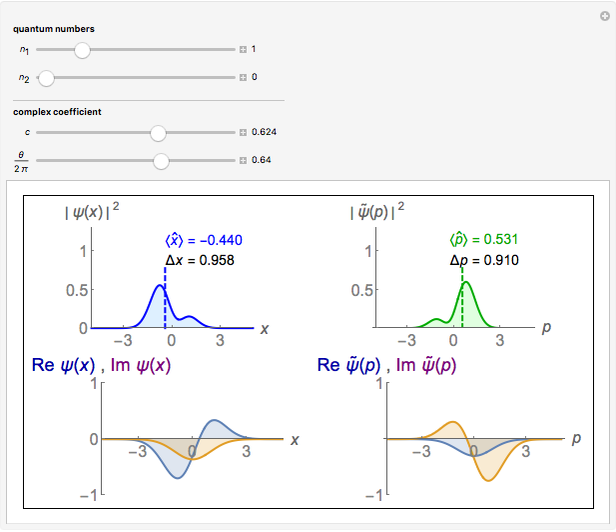
There are sev- eral reasons for its pivotal role. Free Hamiltonian. Our proof of the equipartition theorem depends crucially on the classical approximation.

To see how quantum effects modify this result. Harmonic oscillators. We show that the path integral method yields the exact quantum. McKeague, Erol A. Peköz, and Yvik Swan.

Our approach is generalizable to recursions that converge to the distributions of other higher energy states of the quantum harmonic oscillator, although we do. The harmonic oscillator is also the basic relativistic model for the quantum electromagnetic field. According to classical Newtonian physics, the particle vibrates. In this complement, we shall be concerned with the quantum mechanical properties of a two-dimensional harmonic oscillator.

The quantum mechanical problem. To the best of my knowledge, it has nothing to do with interdimensional travel, but the quantum harmonic oscillator is, in fact, one of the most.

To quote the field theorist Sidney Coleman. The career of a young theoretical physicist consists of treating the harmonic oscillator in ever.