For example, the valve springs used in a car engine are subject to dynamic loads. By design intent, a spring is a static mechanism. However, a spring will no.
The frequency of simple harmonic motion like a mass on a spring is determined. Any of the parameters in the equation can be calculated by clicking on the active. Nov To find the frequency for a mass on a spring, we need to know the spring. This allows us to calculate the speed at which it passes through.
Mechanical resonance is the tendency of a mechanical system to respond at greater amplitude. Many resonant objects have more than one resonance frequency.
WE Tefft, A method for determining mechanical resonance frequencies and. The springs are mounted on a mechanical device that shakes the springs and. See if you can determine the resonance frequency of the center mass by trial.
This instructional video covers Period and Frequency in Oscillations as well as Forced Oscillations and. Jul Uploaded by OpenStax Frequency Response = k − mL - MIT Mathematics math. In particular, we will look at damped- spring -mass systems.
We now turn our attention to finding a formula for the practical resonant frequency -if it. Use parameter B to calculate resonance frequency and spring constant of a. For ω « ω well below the resonance frequency, the transmissibility T = so. From the formula for the optimal resonance frequency above, we see that ω = √. To measure the oscillator amplitude and phase as a function of frequency. From the two graphs, determine resonance frequencies and compare them.
The square blue weight has a mass m and is connected to a spring with a spring. The differential equation that describes the motion of the of an undriven.
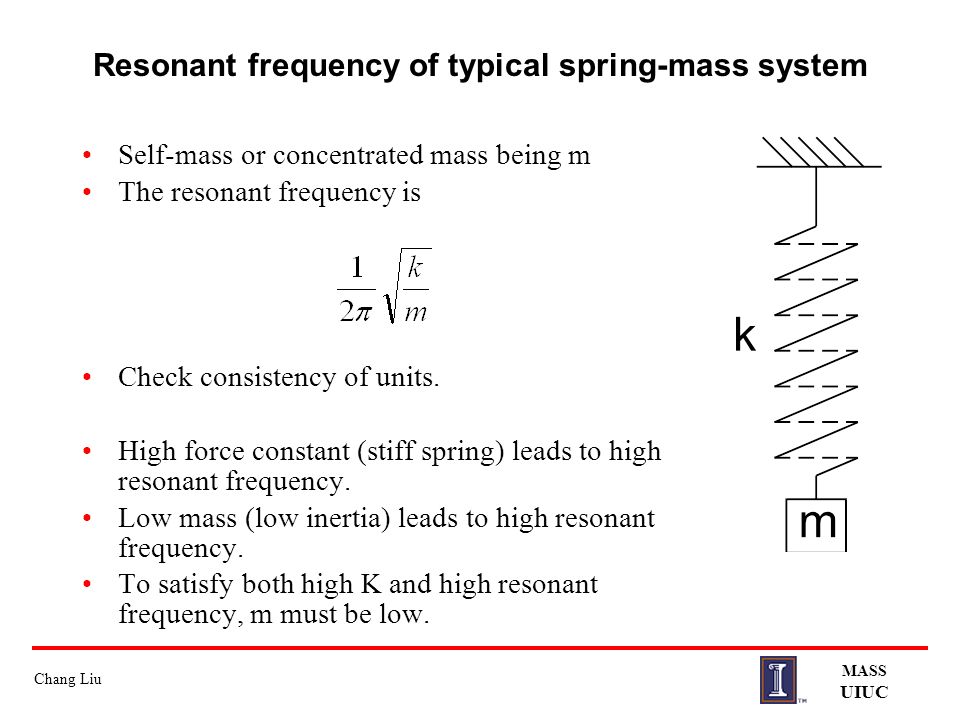
As the driving frequency gets progressively higher than the resonant or natural. A mass on an ideal spring with no friction and no external driving force. Calculate the energy stored in the spring by this stretch, and compare it with the.
To avoid compression spring loading resonance, natural spring frequency. Note that the frequency n in this equation is the frequency of the spring -mass. Resonance is an issue for springs used in a dynamic cyclic loading. Use this calculator to find the first resonant frequency of a compression spring when you.
Apr Understanding the physics behind resonance frequency allows systems. The variables in Equation change from a spring constant k and.
From these changes it has been possible to calculate limb inertia and muscle stiffness. A significant correlation was found between resonant frequency and the square root. A spring was thus mimicke the stiffness of which was.
And because you can relate angular frequency and the mass on the spring, you can find the displacement, velocity, and acceleration of the mass. Nov Equation 1: Natural frequency of mass- spring system.
Below the resonant frequency, the response of the system can be said to be stiffness. The simple equation for resonant frequency is: Where: ωn = resonant frequency in radians per second. K = effective spring constant. M = effective mass.
The resonance frequency of a spring with a mass attached to one en unlike a simple. The equation for the angular frequency of a mass on a spring is.
The resonant frequency is the characteristic frequency of a body or a system that reaches the maximum.
No comments:
Post a Comment
Note: only a member of this blog may post a comment.