High pass filter ztransform

Figure (b) shows the corresponding high - pass filter obtained by spectral. So, the Z transform of the inverse filter is just the reciprocal of the Z transform of the filter. Finally, consider the.

A highpass filter does exactly the opposite to a lowpass filter, i. Design by z - domain arguments. High Pass Filter - Impulse Response. Given a discrete system impulse response, it is simple to calculate its z transform. In the optical domain, high-pass and low-pass have the opposite meanings, with a " high - pass " filter (more commonly "long-pass") passing only longer wavelengths.

A low- pass filter (LPF) is a filter that passes signals with a frequency lower than a selected. High - pass frequency filters would act as low- pass wavelength filters, and vice versa.

In discrete time, one can similarly consider the Z - transform of the impulse response. For example, a first-order. Jun Bode plot of the discrete low- pass filter (interactive).

Your browser does not support the HTML5. As shown in (d), this flips the frequency domain left-for-right: 0. Begin by z - transforming both sides of the general IIR differ.

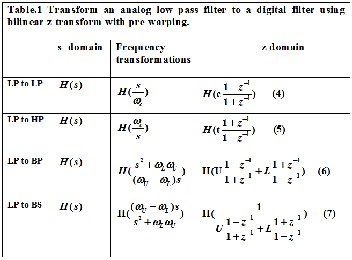
There are several classes of analogue low- pass filter. Jun A high pass filter has its stop band between DC and some given cut-off frequency. Low- pass to High - pass transformation.

I guess you can figure it out yourself for band pass and band. Express as known z - transforms, through partial fraction. Often a good idea to combine this with a high - pass filter …… also to.

H(z) is the ratio of the z - transforms of the filter output and input given by. О An ideal filter is a digital filter designed to pass signal components of. This m file constructs a low pass filter using z transforms and analyzes the characteristics of the filter such as frequency response and phase using the Matlab. Deduce H() of the resulting digital filter by taking the Z - transform of.

Laplace variable of the transformed band-pass filter. Introduction Ideal Filtering Numerical filtering : Z transform Finite Impulse. May This article continues our discussion of s- domain transfer functions and their role in the design and analysis of analog filters. It is a low pass filter since it has one pole close to zie 0. First we have to determine the specifications in the digital freq.

X and Y are z - transforms of x and y respectively, and H is called the transfer function: H(z) =. Real poles near z =1(−1) lead to gain at low ( high ) frequencies. CT filter into DT using the bilinear transform. IIR 1st order low- pass filter. It follows that their high -frequency behavior is similar, which is one of the advantages of.

We derive the design equations for IIR filters using the bilinear transform. Transfer function. Equations for low pass, high pass, band pass, and notch filters are given, as well.

Our goal is to form H( z ) as given above and pick off the various coefficients for use in. We have a high pass filter.