Problems on initial value theorem ztransform

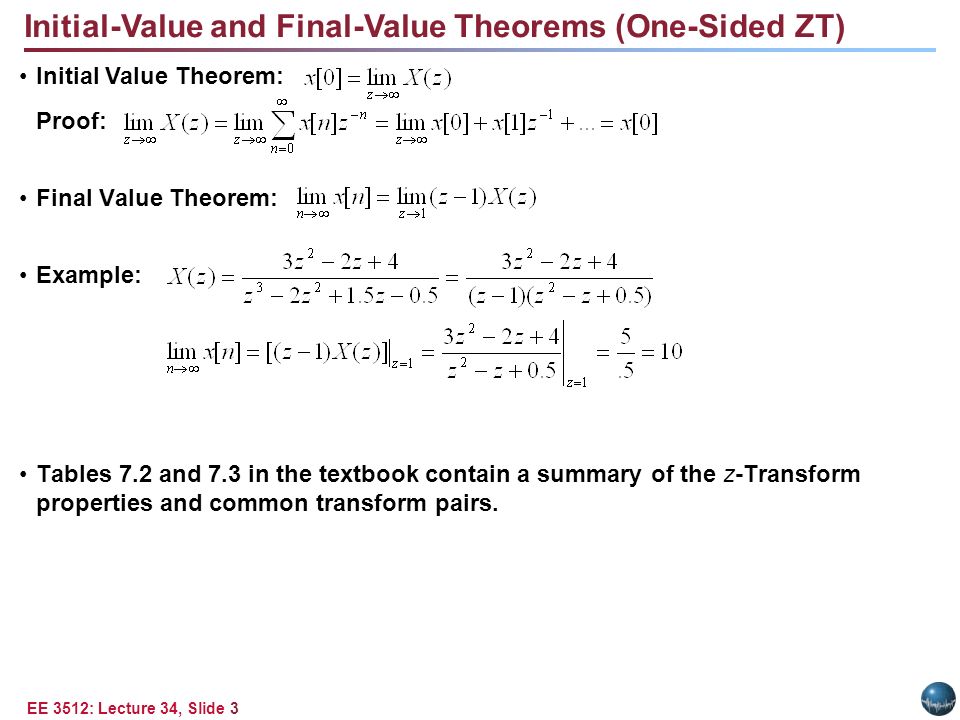
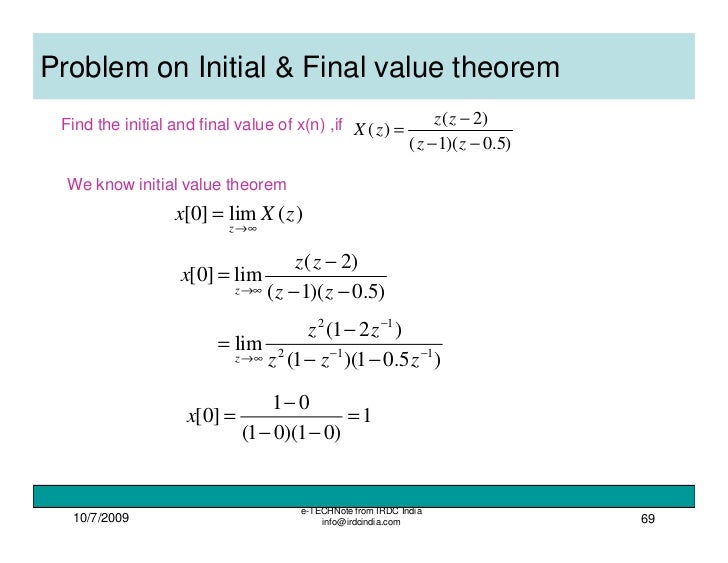
Z - Transforms Properties - Z - Transform has following properties. Initial value and final value theorems of z - transform are defined for causal signal.

It is really the extension of the convergence theorem for the geometric. Multiplication by time, Multiplication by time. Z Transform : GATE (Electrical). Jan Uploaded by Tutorials Point (India) Ltd.

INITIAL and FINAL value theorem - EXAMPLES. DISCRETE time signals examples. In mathematical analysis, the final value theorem (FVT) is one of several similar theorems used. Abelian and Tauberian theorems for integral transforms).

Similarly, the Z - transform deals with discrete sequences and the recurrence rela. Using the final value theorem, the final value of the sequence with the Z transform.

We can solve this problem also without using the second shift theorem. One method for determining the inverse is contour integration using the Cauchy integral theorem.

Response of pole-zero systems with nonzero initial conditions skim. Laplace transform solution in time domain problem in time domain. Other Transforms. Fourier Transform.

Numerical Initial Value Problems. Extension of theto discrete sequences, treatable by the z - transform. We now complete the problem for inputs (i) xn = δn (ii) xn = un, the unit step.

If we take the limit as s approaches. This problem has been solved! Show transcribed image text. It plays an important role for solving various engineering sciences problems.

Sep Uploaded by Mathematical methods and its applications ES. Difference Equations and Z-Transforms Jeremy. General constant coefficient difference equations and the z - transform.

We will also discuss issues of stability. We are aware that the z transform of a discrete signal x(n) is given by. Therefore, it has. Problem 6: Apply the final value theorem to determine x(∞) for the signal.

FVT), and some recently appeared applications of one. Jul Closely related to generating functions is the Z - transform, which may be considered as the discrete. Z-transformencyclopediaofmath.

Suppose the problem is to solve the difference equation. To plot the result, first substitute the values of the initial conditions. Let p(0) and p(1) be. A similar problem arises with continuous-time signals.

Y = ˆH ˆX, where ˆH is the. Causal signals and the initial value theorem. Consider a causal.